IBDP Past Year Exam Questions – Mathematical Induction

Q1.   [M09.P1.TZ2] & [N18.P1]

Prove by mathematical induction  $\sum _{r=1}^n r\left(r!\right)=\left(n+1\right)!–1, n\in {\mathrm{\mathbb{Z}}}^{+}$   .  [8]

Q2.   [N09.P1]

Using mathematical induction, prove that  $\sum _{r=1}^n \left(r+1\right)2^{r–1}=n{2}^n , n\in {\mathrm{\mathbb{Z}}}^{+}$ .  [7]                            .                                                             

Q3.   [M10.P1]

(a)   Consider the following sequence of equations.

                      $$\begin{gathered} 1\times 2=\frac{1}{3}\left(1\times 2\times 3\right) \\ 1\times 2+2\times 3=\frac{1}{3}\left(2\times 3\times 4\right) \\ 1\times 2+2\times 3+3\times 4=\frac{1}{3}\left(3\times 4\times 5\right) \\ ........... \end{gathered}$$

(i)  Formulate a conjecture for the  $n^{th}$ equation in the sequence.

(ii)  Verify your conjecture for  $n=4$ .   [2]

(b)       A sequence of numbers has the $n^{th}$ term given by  $u_n=2^n+3, n\in {\mathrm{\mathbb{Z}}}^{+}$ . Bill conjectures that all members of the sequence are prime numbers. Show that Bill’s conjecture is false. [2]

(c)        Use mathematical induction to prove that  $5\times 7^n+1$ is divisible by 6 for all  $n\in {\mathrm{\mathbb{Z}}}^{+}$ .  [6]

Q4.   [M08.P1]

Use mathematical induction to prove that for $n\in {\mathrm{\mathbb{Z}}}^{+}$ ,

                     $a+ar+a{r}^2+.......+a{r}^{n–1}=\frac{a\left(1–r^n\right)}{1–r}$ .         [7]

Q5.   [M11.P2] & [M18.P1]

Prove by mathematical induction that, for $n\in {\mathrm{\mathbb{Z}}}^{+}$ ,

$1+2\left(\frac{1}{2}\right)+3{\left(\frac{1}{2}\right)}^2+4{\left(\frac{1}{2}\right)}^3+........+n{\left(\frac{1}{2}\right)}^{n–1}=4–\frac{n+2}{2^{n–1}}$ .   [8]

Q6.   [M17.P1]

Use the method of mathematical induction to prove that $4^n+15n–1$ is divisible by  $9$ for  $n\in {\mathrm{\mathbb{Z}}}^{+}$ .  [6]

Q7.   [M13.P2]

Use the method of mathematical induction to prove that $5^{2n}–24n–1$ is divisible by $576$  for all  $n\in {\mathrm{\mathbb{Z}}}^{+}$ . [7]

Q8.   [M14.P2]

Prove by mathematical induction that $7^{8n+3}+2$  ,  $n\in \mathrm{\mathbb{N}}$ ,  is divisible by $5$ . [8]

Q9.   [N16.P1]

Q10.   [M15.P1]

Q11.   [N14.P1]

Use mathematical induction to prove that  $\left(2n\right)!\ge 2^n{\left(n!\right)}^2 , n\in {\mathrm{\mathbb{Z}}}^{+}$ .   [7]

Q12.  [M16.P1.TZ1]

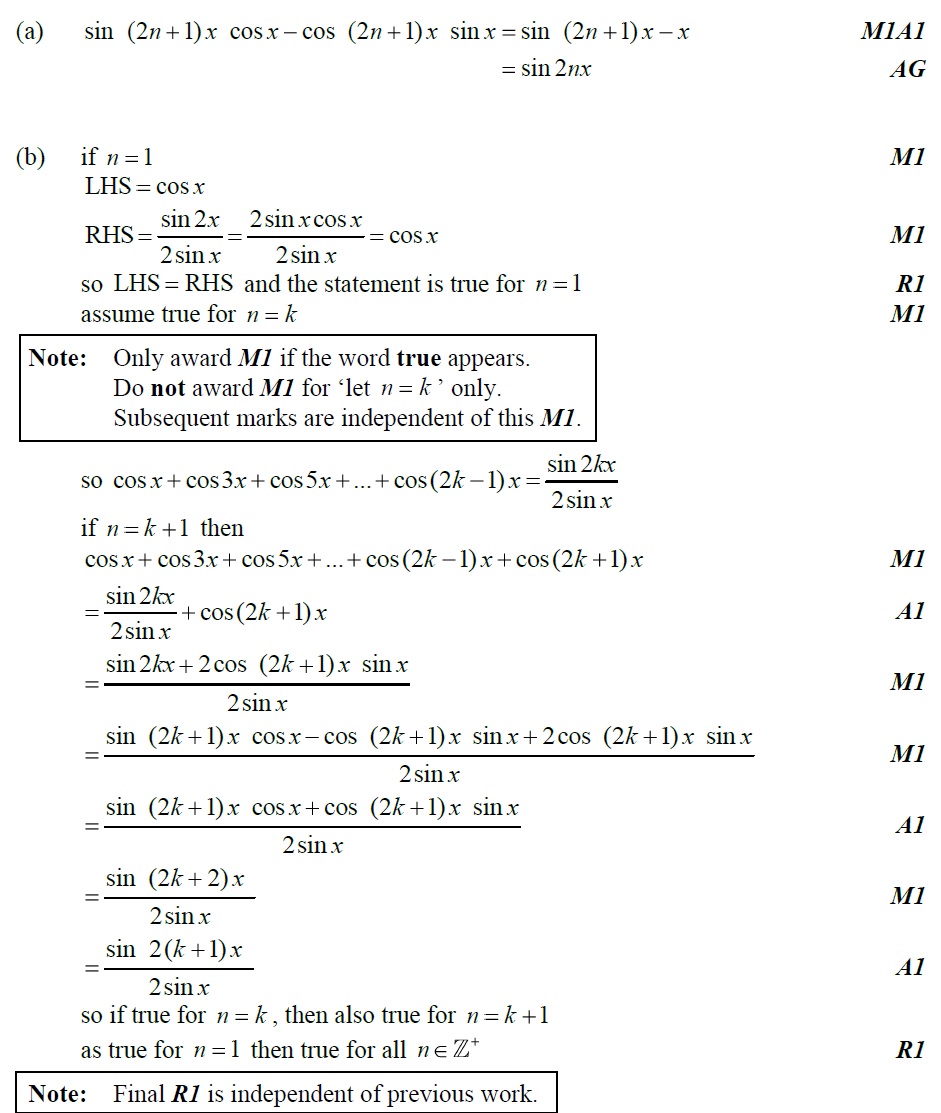
Q13.   [M10.P1]

(a)        Show that  $\sin 2nx=\sin \left(\left(2n+1\right)x\right)\cos x–\cos \left(\left(2n+1\right)x\right)\sin x$ .

(b)        Hence prove, by induction, that                                                              

                $\cos x+\cos 3x+\cos 5x+........\cos \left(\left(2n–1\right)x\right)=\frac{\sin 2nx}{2\sin x}$ ,

             for all $n\in {\mathrm{\mathbb{Z}}}^{+} , \sin x\ne 0$ .

Q14.   [N17.P1]

Consider the function  $f_n\left(x\right)=\left(\cos 2x\right)\left(\cos 4x\right).....\left(\cos 2^{n}x\right) , n\in {\mathrm{\mathbb{Z}}}^{+}$  

(a)        Determine whether $f_n$ is an odd or even function, justify your answer.    [2]

(b)        By using mathematical induction, prove that                                                     

                    $f_n\left(x\right)=\frac{\sin 2^{n+1}x}{2^n\sin 2x} , x\ne \frac{m\mathrm{\pi }}{2}$   where  $m\in \mathrm{\mathbb{Z}}$ .   [8]